WHY TEACH THIS?
Optimisation, or finding the best possible solution to a problem, is an important real-life application of mathematics. We often want to minimise (find the cheapest or quickest way to do something) or maximise (find how to get the most of something). Calculating beforehand, rather than guessing-and-hoping, can save time, effort or money.
Often we use common sense and intuition to decide on a course of action, and frequently that is the best thing to do. But sometimes mathematics can help us to make better decisions. If you need to travel from one location to another, you might want to find the shortest or fastest route.
Even if a shorter route is only slightly shorter, if you are going to be making the journey repeatedly it could add up to a significant saving. Sometimes it is obvious simply by looking at a map which route is going to be the best, but at other times mathematical calculations can help. In this lesson, students have to work out which is the shortest way to get from one location to another on a map. They will need to use their knowledge of scale and perhaps Pythagoras’s theorem, and they will also need to be systematic, well-organised and decide how to communicate their conclusions clearly and convincingly.
STARTER ACTIVITY
Project or draw the picture below on the board:
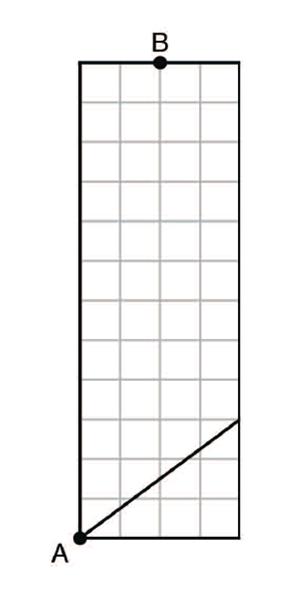
Q. Suppose I want to get from A to
B. The dark lines are roads and the faint lines are just a grid showing the distance. Let’s say that each little square is 100 metres by 100 metres. Someone come and show us with your finger one route from A to B. Now someone else come and show us another possible route. You can only go along the roads!
The two possible routes are shown in blue and red below.

Q. Which is the quicker route or are they the same? Why?
The red route probably looks longer, but can learners justify why it must be longer than the blue route? they could do this by counting the sides of the squares. this involves deciding how long the slanting part of the red route is. it is the hypotenuse of a 3-4-5 rightangled triangle, so this is a good opportunity to review Pythagoras’s theorem, which learners may need to use later in this lesson. so the blue route is 14 and the red route is 16, so blue wins.
It is also possible to see this without doing any calculations. the horizontal sections of the two routes are equal, so can be ignored. and if the vertical parts in common are also removed we can see that the choice is between a vertical blue section or a slanting red section:
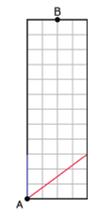
Clearly the blue part is shorter, since it goes directly up, so blue wins. One advantage of this argument is that we can see that it doesn’t depend on the exact dimensions of the rectangle. another advantage is that we can work it out even if we don’t know Pythagoras’ theorem!
Q. Now suppose I want to get to point C instead. What is the shortest way to get there from A?
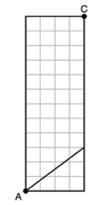
Again there are two possible routes, and this time the red route is shorter.

Removing the common vertical sections shows that the choice is between the hypotenuse of a right-angled triangle and the sum of the two legs, and the hypotenuse is clearly shorter. (the longest side of a triangle is always shorter than the sum of the other two sides.)

Q. What do you think will happen if we want to go to point D?

Learners will probably guess that the two routes will be equal, and they can check this by calculation (both are 15 units long).
MAIN ACTIVITY
Q. Now you can see more of the map. Choose a letter and find the different possible routes from A to your letter. Then try another letter
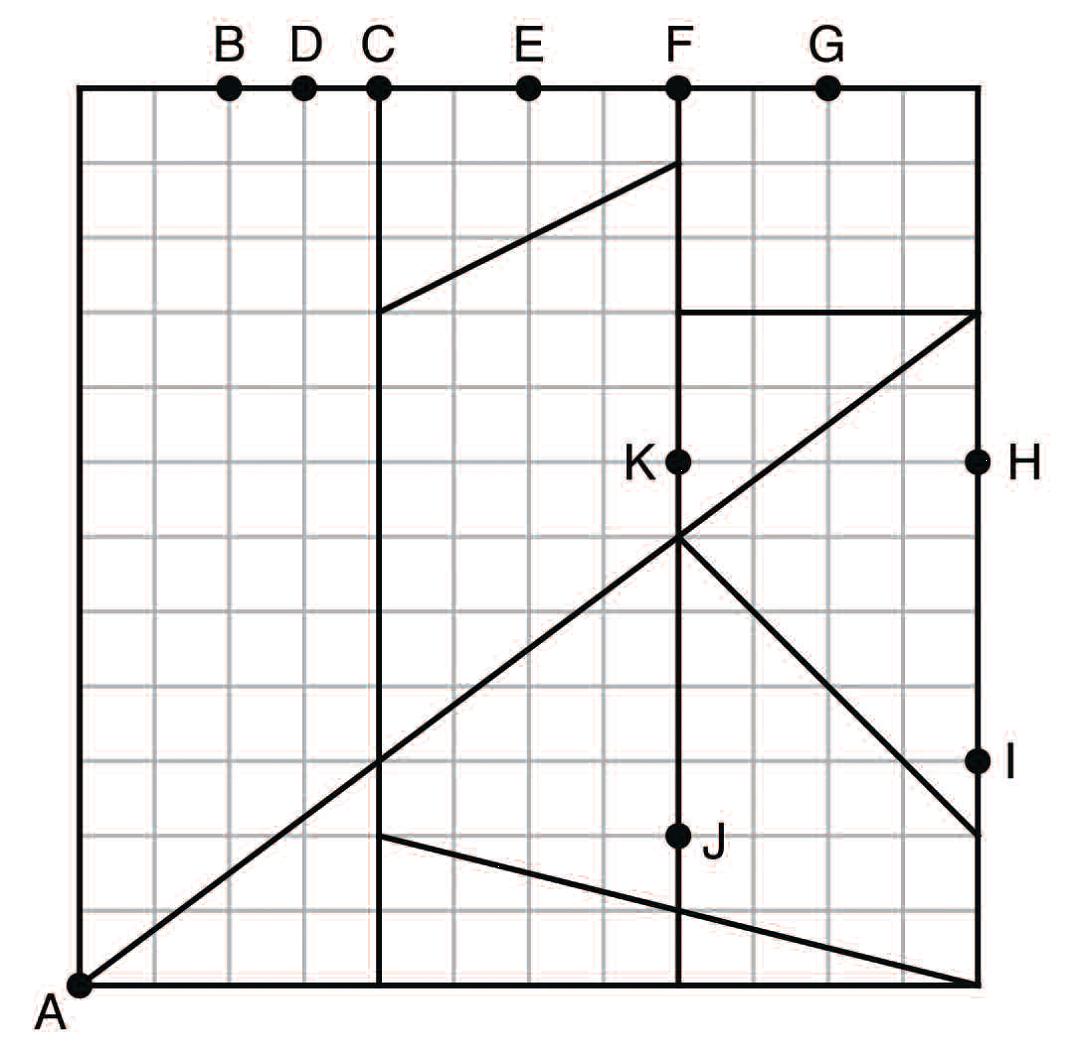
. There is lots to explore here. Learners can use a mixture of calculation and argument (e.g. comparing common lengths) to justify their answers. their judgment will tell them which routes are not worth considering, because they are obviously longer. students may be surprised that routes involving “diagonals” are not necessarily shorter!
Students will need to be well organised in recording their results so that they don’t get muddled up about which two locations their route is between. When they find a shorter route to one location this could affect their previous results to other locations, and they might need to go back and modify their answers.
Students might like to estimate in advance the order of the locations B-K according to their distance from a and then see how accurate they were. students who complete the task could consider starting from a different letter than a.
DISCUSSION
You could conclude the lesson with a plenary in which students talk about what they have found out and learned.
Q. What did you find out? Which ones were hardest to work out? Which ones could you decide without doing any calculations? When did you need Pythagoras’ theorem? Did anything surprise you?
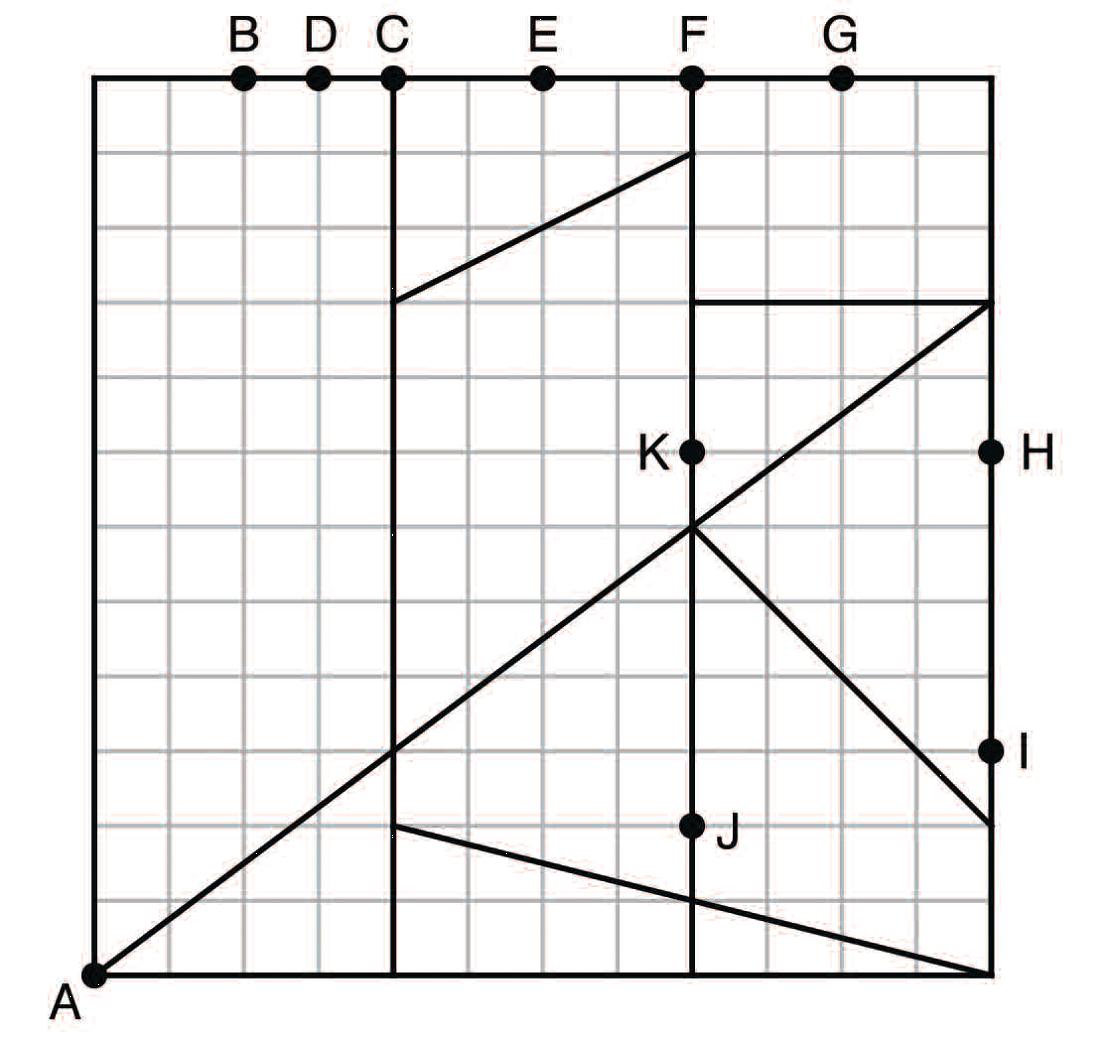
The order of locations according to their distance from A is
J < K < B = C < D = I < E = F < H < G,
and students might well find this surprising and counter-intuitive. It is certainly very different from the direct distance “as the crow flies” from A to these locations.
ABOUT OUR EXPERT
Colin Foster is an Assistant Professor in mathematics education in the School of Education at the University of Nottingham. He has written many books and articles for mathematics teachers (see www.foster77.co.uk).








